Conceptual principles of the system (emergent)
information theory
& its application for the cognitive modelling of the active objects (entities)
Eugene V. Lutsenko, Dr.Sc.(Tech.)
E-mail: lutsenko@fromru.com; URL: http://www.Lc.narod.ru
In the classical Schennon information theory based on the generalisation of the results of Boltsman, Nyquist and Hartley, the very notion of information is defined proceeding from the theoretical-set (=set-theoretical) and combinatory representations on the basis of the analysis of behaviour of a classic macroobject which is capable of changing only into the well fixed alternative reduced states. But the quantum entities (objects) and the complex reflexive systems can be found at a time in two and even more alternative for the classic entities (objects) states. Such states can be called the mixed ones. Thus the pattern of after-effects has been observed which cannot be interpreted merely as the common total sum of the after-effects of the alternative options, i.e. the pattern that surprisingly resembles the quantum physical phenomenon and which is called the probability density interference. This phenomenon, by far having the system (emergent) pattern, is offered to call “the option after-effects interference”. The generalisation of the Hartley – Schennon information theory has been proposed (advanced, suggested) by way of considering the quantum and active entities as the objects on the basis of the analysis of which the basic conception of the information itself has been developed (shaped). The information theory generalised in this way is suggested to call the system or the emergent information theory (it would be also to aptly call it the quantum one but this term has already been used in another sense). The major distinction of the emergent information theory from the classical one is the account of the properties of the systematic character both of the fundamental and universal properties of all the objects (entities) at the level of the information cenception itself, and not just in what follows (in the subsequent text), as in the classical theory.
It will suffice to consider the quantum generalisation of the Hartley theory, for the way the Schennon theory has been derived from the Hartley theory is widely known The system (emergent) generalisation of the Hartley formula has been offered for an amount of information:
|
|
(1) |
where, W – the number of the pure (classical) state
of the system; ![]() – the
combinations “of W on m”of the classical states.
– the
combinations “of W on m”of the classical states.
Since =W, then at M=1 the expression above takes the classical form of the Hartley’s formula, i.e. the conformity
principle is being implemented which is binding for the more general theory.
At M=W the expression (1) takes the form of:
|
|
(2) |
The additional information received as a result of an object (entity) behaviour in the system (emergent) information theory (SIT) is actually the information on the multitude of all its possible states, as a system, an element of which is the object (entity) in some given state.
The numerical computations and the analytical calculations show that as the number of elements in the system increases, the share of the system (emergent) information in the behaviour of its elements also enhances increasingly. This property of the systems found by us is offered to call “The Law of the Emergentability Increase”.
The system generalisation of the classical A. Kharkevich formula available for an amount of information has acquired the following form:
|
|
(3) |
where, φ – the Hartley emergentability ratio (the level of the system organisation of an entity (object) having W pure states):
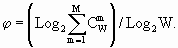
The formula (3) accounts for both the interaction between the criteria (factors) and the future, including the aim-intended states of a control object, and the power of the multitude of the future states. Besides, it unites the possibilities of the integral and discrete description of the objects that is considered to be the basis of the formalisation of a sense (meaning), as well as meets the principle of conformity, i.e. changes into the Hartley formula in the extreme case, when for every class (object state) there is one criterion (factor), and for every criterion – one class, and these classes (and also the criteria) are equally probable (equiprobable).
In this case the factors are understood as the controlling ones, i.e. operations of the control system, the environmental factors, the factors which characterise the current and the past states of a control object, including its activity and reflectivity (values, evaluations and self-appraisals, the systems, motivation, modelling, taking and
-----------------------------------------------------
fulfilling the decisions). All this makes the formula (3) to be optimum as to the formulated criteria for the purpose of constructing the substantial, informative models of the active control objects and for using for the synthesis of the reflexive computer-aided control systems/automatic control systems (CAC/ACS) by the active systems. The author worked out the universal cognitive analytical system “Aidos”, in which the algorithms of the ten basic cognitive operations of the system analysis, the algorithms of the cluster-constructive and cognitive analysis, fuzzy logic and cognitive graphics have been implemented; the original visualisation of the results of the data intelligent analysis (fuzzy graphs) have been afforded.
The generalised (generic) decision table have been generated in which the inputs (factors) and outputs (the future states of the control active object (CAO) are linked together not with the help of the classical (Aristotel) implications taking only the meaning of “Truth” anf “False”, but with the help of different meanings of the truth expressed in bytes and taking the meanings from the theoretically maximum allowable positive (“The maximum degree of the truth”) to the theoretically unrestricted negative (The degree of falsehood). The synthesis of the generic decision tables for the different application domains is carried out directly on the basis of the empirical initial data; on the basis of these tables the direct and reverse probable (fuzzy) logic reasonings on the non-classic diagrams with the different estimated meanings of the truthare produced, thus being (the synthes) the generalisation of the classical implications. In this direct reasonings some factors are considered as the preconditions, and the future states of CAO - as the conclusions, and in the reverse ones – vice versa. The degree of the truth of i-precondition is a mere quantity of information I contained in the precondition on coming of the j-future state of the active control object (expression 3 of the SIT). The Euclidean space as the measure of likeness of an object with a class, a class with another class and an attribute with another attribute is not acceptible because the informative semantic space, generally speaking, is not orthonormal, as to the Makhalonobis space it is also not applicable, for it measures the distance starting from the centrode but not between the vectors, therefore on the basis of the Nieman-Pirson lemma it is advisable to use the total amount of information. If there are a few preconditions, then the degree of the truth of incoming of the j-state CAO equals to the total amount of information available in them about it (4, 5):
|
|
(4) |
In the expression (4) the parentheses denote the scalar product. In the coordinates form this expression (term) has the following appearance:
|
|
(5) |
where, – ![]() a vector of a j-state of the control object , which coordinates can
be estimated in the informative semantic space according to the expression
(3);
a vector of a j-state of the control object , which coordinates can
be estimated in the informative semantic space according to the expression
(3); ![]() – the
Boolean vector of the application domain state having all the types of
factors which characterise the control object, the possible control
effects and the environment (array-locator), i.e.:
– the
Boolean vector of the application domain state having all the types of
factors which characterise the control object, the possible control
effects and the environment (array-locator), i.e.:
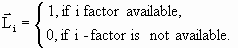
The direct probable logic reasonings allow to foresee the degree of the truth of incoming of an event - as per the existing factors, and the reverse ones – as per the given state to restore the degree of necessity and the degree of the undesirability of each factor to approach this state, i.e. to take decisions in selecting of the control effects on the CAO, which are optimum to transmit it to the given target state. It is pertinent to note that the offered model based on the information theory provides the automated formation of the system of the fuzzy rules on the content of the input data as the combination of the Zade-Cosco fuzzy logic with the Kohonen neuron network. It is of basic importance to know that the qualitative change of a model by adding the new classes to the model does not decrease the truth of understanding of the already formed classes. Besides, when comparing the object to be understood with each new class not only the features which an object has are taken into account, but those missing, therefore with the help of the model proposed only those objects can be correctly identified, which features form the sets one of which is the subset of another (the same as in the K. Fukshima Neokognitrone).
Conclusions and suggestions: the mathematical model, algorithms of the basic cognitive operations, the instruments (tools) used by them (the general-purpose cognitive analytical system “Aidos”) provide the synthesis of the reflexive CAC/ACS by the active objects. Experience of the efficient usage of the theory considered in this paper, its provisions and the tools for controlling the technologies, solving the CAC/ACS tasks for training the skilled specialists, for forecasting the development of the stock exchange (market) segments, of the sociological and polytological researches has been gained for development and application of the tests on a profession (speciality), as well as in a number of other domains. It is the precondition to. extend the field of its usage by way of creation of the multiagent distributed systems of observation and accumulation of knowledge in Internet and the integration of the offered know-hows (technologies) with the expert systems based on the fuzzy logic.

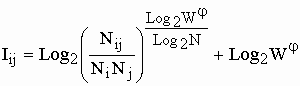
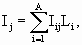 ,
,